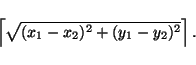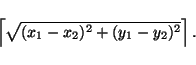Next: MINIMUM GENERALIZED STEINER NETWORK
Up: Spanning Trees
Previous: MINIMUM STEINER TREE
Index
- INSTANCE:
Set
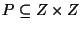 of points in the plane.
of points in the plane.
- SOLUTION:
A finite set of Steiner points, i.e.,
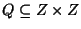 .
.
- MEASURE:
The total weight of the minimum spanning tree for the vertex set
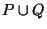 ,
where the weight of an edge
,
where the weight of an edge
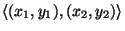 is the discretized
Euclidean length
is the discretized
Euclidean length
- Good News:
Admits a PTAS [33].
- Comment:
Admits a PTAS for any geometric space of constant
dimension d, for example in the rectilinear metric [34].
MINIMUM STEINER TREES WITH OBSTACLES, the problem where a polygonally
bounded region R is given in the input, and the Steiner tree has to lie
inside R, admits a FPTAS under some restrictions of the input
[409].
- Garey and Johnson: ND13
Viggo Kann
2000-03-20